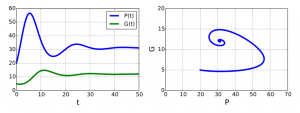
So the number of rainbowfish will grow fast from the initial 20 to about 55 in the first week. Because of the plentyfull rainbowfish, the number of gouramis will triple in a week and a half. The gouramis will eat more and more of the rainbowfish, so the number of rainbowfish will decrease again to about 25 after two weeks. With fewer rainbowfish, the number of gouramis decreases again, giving the rainbowfish population the chance to grow again. And so on, and so on. The increases and decreases of both populations become smaller in time, and after a month and a half, both populations stabilize to approximately 31 rainbowfish and 12 gouramis.