Torsion: Angle of Twist

Course subject(s) 3. Torsion

Derivation of the angle of twist for a thin-walled closed section shaft requires the application of energy methods beyond the scope of the current course. However, it is still necessary to be able to calculate this angle of twist in order to meet the learning objectives of this course (solve statically indeterminate problems containing thin-walled shafts). As a result, the following equation for angle of twist in a uniform thin-walled torsional shaft is provided to you:

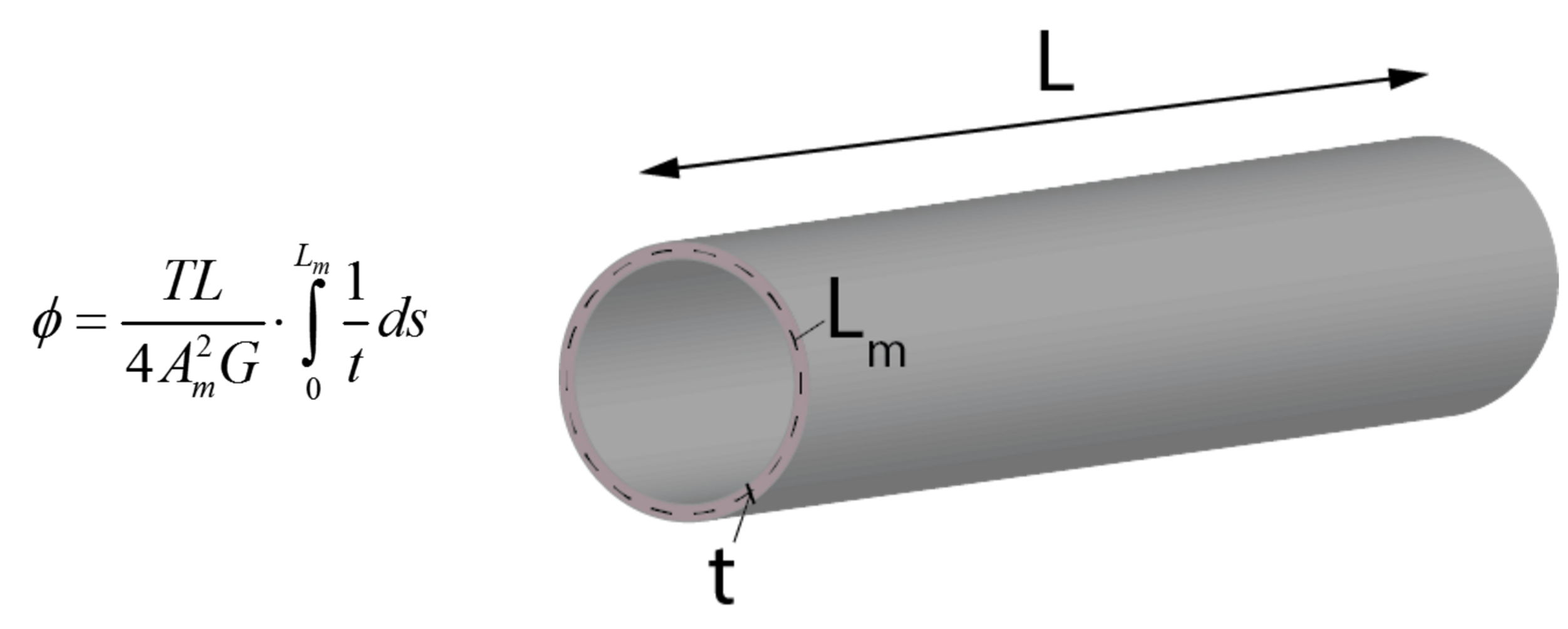
Here, Phi is the angle of twist, A_m is the area enclosed by the median line of the cross-section, L_m is the length (perimeter) of the median line, and ds is a differential increment along the median line. Please note that the equation contains an integral that needs to be performed around the preimeter of the cross-section median line.

Aerospace Mechanics of Materials by TU Delft OpenCourseWare is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License.
Based on a work at https://ocw.tudelft.nl/courses/aerospace-mechancis-of-materials/.